An Eigenanalysis of Angle-Based Deformation Energies
Abstract
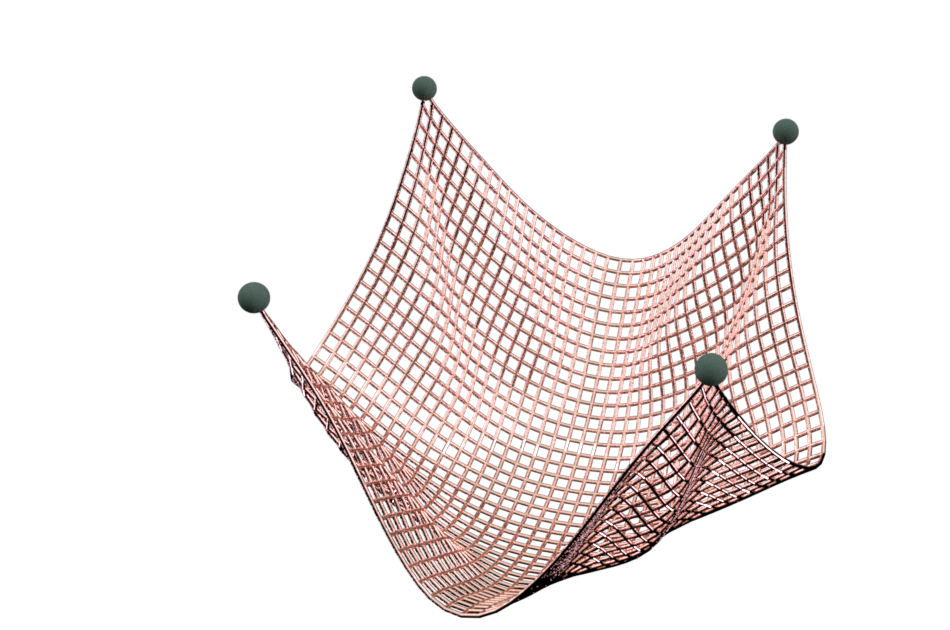
Angle-based energies appear in numerous physics-based simulation models, including thin-shell bending and isotropic elastic strands. We present a generic analysis of these energies that allows us to analytically filter the negative eigenvalues of the second derivative (Hessian), which is critical for stable, implicit time integration. While these energies are usually formulated in terms of angles and positions, we propose an abstract edge stencil that succinctly parameterizes the edge deformation, and allows us to derive generic, closed-form analytical expressions for the energy eigensystems. The resultant eigenvectors have straightforward geometric interpretations.We demonstrate that our method is readily applicable to a variety of 2D and 3D angle-based elastic energies, including both cloth and strands, and is up to 7x faster than numerical eigendecomposition.
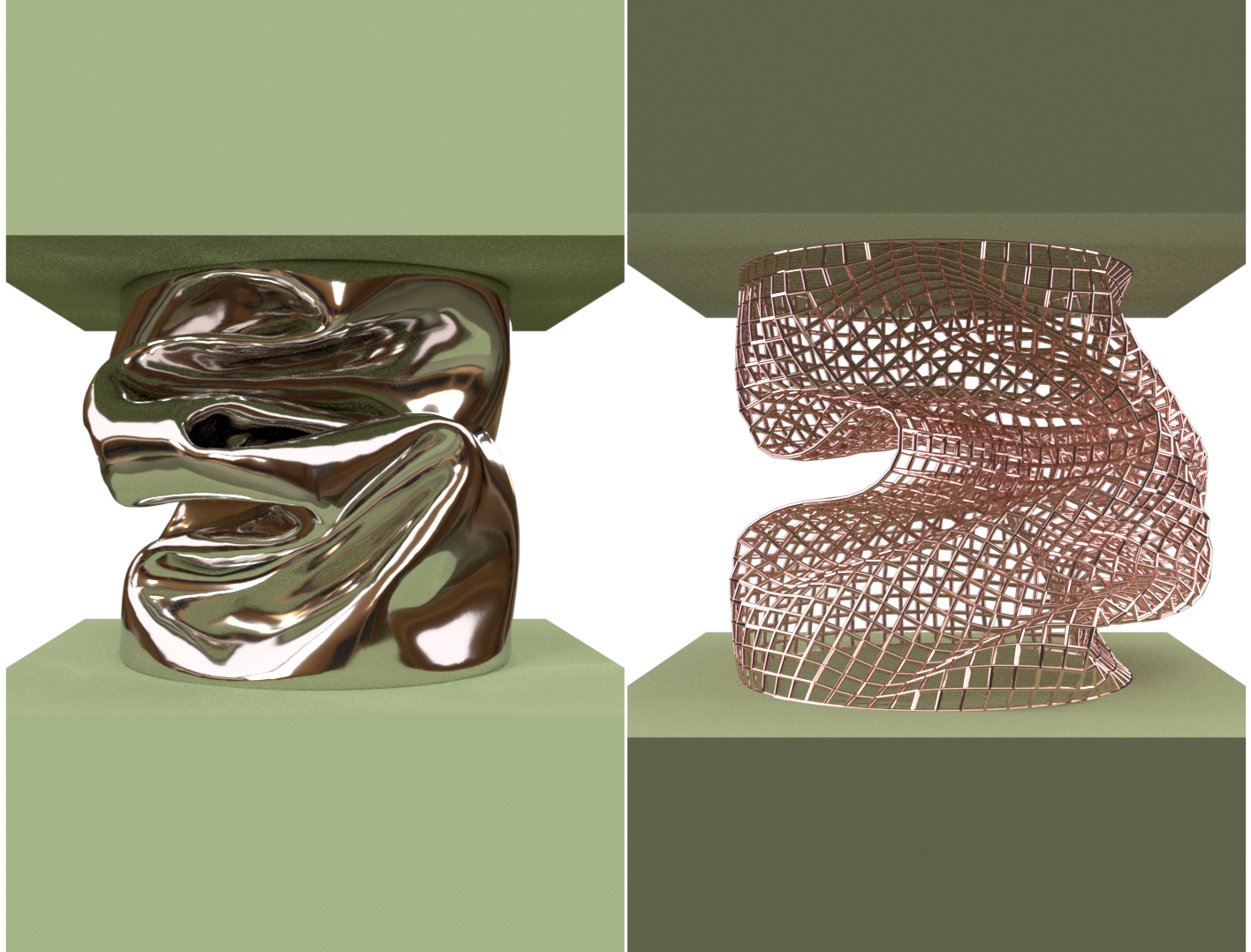
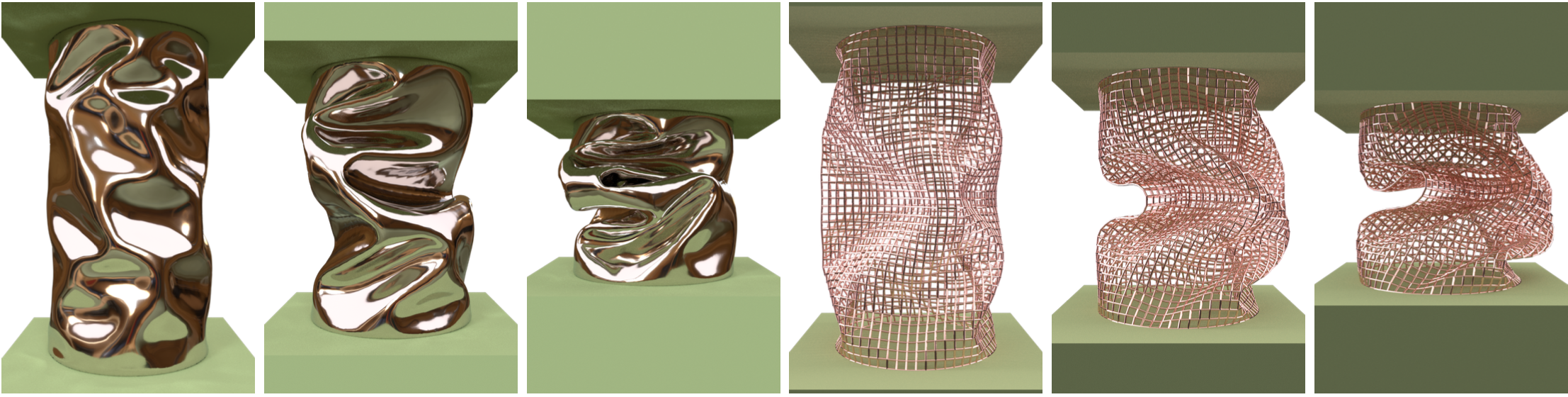
Examples